Introduction
There are a lot of publication on greenhouse effect. However the strong feeling exist that “something is rotten in the state of Denmark”. The theory and formulas behind greenhouse effect strongly contradict to common sense and observations.
Here I am trying to show such contradictions on the example of planet Venus.
Greenhouse Effect
In simple words greenhouse effect is the following – part of radiant heat emitted by the planet's surface radiated back, increasing temperature of the surface.
Solar Constant
In theory, accepted by mainstream science, the blackbody emit radiation according to Stefan-Boltzmann law which describes power density as function of temperature:
$$\Large P_S = \sigma T^4 \tag{1}$$where \(\sigma\) is Stefan-Boltzmann constant equals to 5.67×10-8 and \(T\) is the temperature, which equals to 5800K for the Sun. After calculation:
$$\Large P_S \approx 6.29\times 10^7 \quad W \cdot m^{-2} \tag{2}$$This is the power density at the surface of the Sun. In the vicinity of some planet the power will be spread over the surface of the sphere with radius equals to the average distance from Sun to planet. Such power density called solar constant for the planet.
$$\Large 4 \pi R_S^2 P_S = 4 \pi R_P^2 K_S \tag{3}$$We are looking at the planet Venus. The mean radius of the Sun equals to 696021 km and mean radius of Venus orbit is 108,208,000 km. So, the solar constant for Venus equals to 2604 W·m-2.
Temperature
Some part of the energy will be reflected back to space. The reflected part over incoming energy called albedo. Albedo of Venus equals to 0.75. The rest will heat the surface.

Fig.1 Incoming energy equals to emitted energy.
Incoming energy should be equal to radiated energy. Incoming energy per unit area multiplied by planet's projection, but the whole surface of sphere will radiate energy:
$$\Large E_{IN}=E_{OUT} \tag{4}$$ $$\Large (\alpha -1)K_S \pi R^2 = \sigma T^4 4 \pi R^2 \tag{5}$$and:
$$\Large T=\sqrt[4]{(1-α)K_S \over {4 \sigma}} \tag{6}$$Result for temperature is 231.5K (-41.7C).
Observed temperature of Venus equals to 735K (462C). The difference attributed to strong greenhouse effect. We are going to take a look at this effect.
Greenhouse Once Again
Simplified model of greenhouse effect shown below.
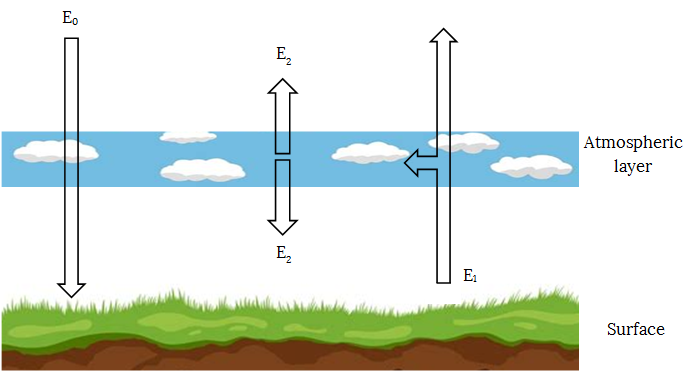
Fig.2 Simple greenhouse model.
On the figure above \(E_0\) is incoming energy from the Sun. The energy spectrum is out of atmospheric absorption band and this energy coming through atmosphere unaffected. \(E_1\) is the energy emitted by planet. Part of this energy \( \beta E_1\) absorbed by atmosphere and the rest \( (1-\beta )E_1\) returned back to space. Energy \(E_2\) emitted by atmosphere equally in both directions.
Despite of the simplicity this model works for Earth. By “work” I mean the fact that calculations followed from this model are used as a proof of Earth's greenhouse effect.
Balances
Now it's time for simple math. Don't worry, not a rocket science. Since everything in equilibrium, “energy in” always equals to “energy out”. Here are the balances:
• Space balance:
$$\Large E_0 = E_2 + (1-\beta )E_1 \tag{7}$$• Atmosphere balance:
$$\Large \beta E_1 = 2E_2 \tag{8}$$• Surface balance:
$$\Large E_0 + E_2 = E_1 \tag{9}$$From (8) follows that:
$$\Large E_2 = {\beta \over 2} E_1 \tag{10}$$Substituting to (9) gives:
$$\Large E_0 + {\beta \over 2}E_1 = E_1 \tag{11}$$And:
$$\Large E_1 = {{2E_0} \over {2 - \beta}} \tag{12}$$ $$\Large E_2 = {{\beta E_0} \over {2 - \beta}} \tag{13}$$Maximum temperature rise will happen when all energy radiated by planet absorbed by atmosphere (\(\beta = 1\)). There is a lot of carbon dioxide, isn’t it?
Then energy radiated by surface will be:
$$\Large E_1 = 2E_0 = 2K_S(1-\alpha) = 1302 \quad W \cdot m^{-2} \tag{14}$$Here we come to the temperature part using (1):
$$\Large T = \sqrt[4]{E_1 \over \sigma} = 389K = 116C\tag{15}$$As was mentioned before the temperature of the Venus equals to 735K (462C). We are missing 350 degrees.
Greenhouse effect just could not afford any more.
Conclusion
Greenhouse effect could not be responsible for the high surface temperature of Venus.